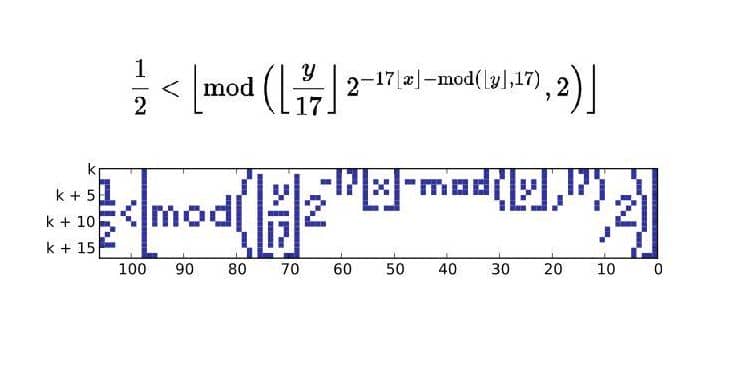
In Physics, everything can be described by an equation. These equations can then further describe the shape of lines, curves, surfaces, and almost anything you can think of. In fact, there is hardly anything that cannot be described by an equation. This leads to another question: Can there be a mathematical equation that can describe itself? The answer is, yes. The equation is called Tupper’s Self-Referential Formula and it looks like this:
Tupper's self-referential formula is a formula that visually represents itself when graphed at a specific location in the (x, y) plane. pic.twitter.com/wAUVahJ9Dq
— Fermat's Library (@fermatslibrary) February 4, 2018
The upper part of the image is the equation itself and the bottom part is the graph of the same equation. Both the graph and the equation are just the same. This equation cheats a little too. If you notice closely, the y-axis of the graph is beginning at ‘k’. Here k is a 543-digit number, so this graph is actually very high on the y-axis.
The Tupper’s Self-Referential Formula is not only describing itself, rather it is describing everything. The graph created by the formula features every possible 106 by 17-pixel grid which is arranged one after another along the y-axis. To find a specific grid, all you need to do is to travel up the axis. This video will explain a little more background and context on Tupper’s Self-Referential Formula.